8.2.1 Parameterization of the Dispersion Relations

  Each set of calibration images is processed to provide analytic relations between wavelength and pixel position in low-dispersion SI space. The derivation of these dispersion relations is a multi-step process. First, the pixel locations of the Pt-Ne emission lines in a reference low-dispersion WAVECAL image are measured interactively and combined with laboratory values for the wavelength of each emission line (stored in a line library). This analysis is performed using the IRAF task identify which generates a dispersion solution that is a one-dimensional fitted function (Chebyshev polynomial) of wavelength versus pixel number. The next step involves the use of the IRAF task reidentify which maps the reference-image Chebyshev solution derived from the identify step to an ensemble of images. The final dispersion solution is averaged from several hundred individual solutions output from reidentify and consists of a starting wavelength and wavelength increment per pixel. The line libraries are based on the Pt-Ne line positions measured by Reader et al., (1990) at the National Institute of Standards and Technology (NIST). Figure 8.1 shows low-dispersion WAVECAL spectra for the LWP/LWR and SWP cameras and indicates the features included in the low-dispersion libraries. Table 8.1 contains listings of the low-dispersion line libraries. The line positions for all cameras, and therefore the dispersion solutions, are expressed in vacuum wavelengths.

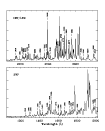Figure 8.1:  Short and long wavelength low-dispersion WAVECAL spectra. Pt-Ne features included in the low-dispersion line libraries are marked.

 

 

Short Wavelength Long Wavelength

Camera (Å) Cameras (Å)

1248.61 1913.23

1289.95 1990.58

1302.79 2037.12

1380.49 2050.05

1403.90 2085.26

1429.52 2129.31

1482.83 2144.92

1509.29 2263.42

1524.73 2357.83

1574.31 2440.80

1604.01 2468.15

1621.65 2487.92

1669.23 2539.97

1723.13 2677.94

1736.52 2734.77

1753.83 2772.48

1802.94 2792.84

1971.54 2831.13

1990.58 2876.48 2930.65 3094.90 3140.30 3219.12 3324.69 3346.42

Because the geometric correction and resampling step of the image processing rotates the low-dispersion images so that the dispersion direction is parallel to the horizontal or x-axis of the low-dispersion SI, the terms of the dispersion solutions for the two image dimensions are completely decoupled. The parameterization of the dispersion solutions is as follows:

$$x = A_1 + A_2\lambda$$

y = B₁

where x and y are the image pixel locations and A₁, A₂, and B₁ are the dispersion constants. For a low-dispersion SI, the y dimension of the image contains spatial information only. In this case the B₁ coefficient simply gives the image line number at which the spectrum centroid is located.