


Next: 3.9 Choice of Long-Wavelength Camera
Up: 3 Pre-Observation Preparations
Previous: 3.7 Moving Targets
It is occasionally important to know the astronomical orientation of the
spectrograph apertures, as in observations of nebulae, galaxies, and close
double stars. Equations for the spacecraft roll angle and the orientation
angles of the apertures are given in an article by Schiffer (1980a) and are
reprinted below. The orientation, especially with respect to the spectrum, is
not always intuitively obvious. As a visual aid in finding the orientation of
the apertures, formats of the FES field and the cameras are depicted and
discussed below.
Since the FES and spectrographs are fixed within the spacecraft, the FES
coordinate system (labelled X and Y) is defined relative to the inertial axes of
the spacecraft (see Figure 3.3). These coordinates are used to specify the
locations of the apertures, guide stars, and so forth, in the telescope field of
view.
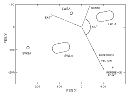 Figure 3.3: Aperture plate as
viewed by FES
No. 2. The locations of the apertures and the reference point are shown as
seen by FES No.2. The direction of the reference vector (the direction of
stellar motion for a positive pitch slew) is indicated. The X and Y FES
coordinates are given in FES fine units (1 unit = 0.26 arcseconds). The North
and East directions are shown for a spacecraft roll of 130 degrees.
Figure 3.3: Aperture plate as
viewed by FES
No. 2. The locations of the apertures and the reference point are shown as
seen by FES No.2. The direction of the reference vector (the direction of
stellar motion for a positive pitch slew) is indicated. The X and Y FES
coordinates are given in FES fine units (1 unit = 0.26 arcseconds). The North
and East directions are shown for a spacecraft roll of 130 degrees.
The aperture and spectrum orientation in the plane of the sky is a function of
the spacecraft roll angle. The spacecraft roll is defined as the angle,
in the plane of the sky, between north and a reference vector (see below)
pointing to the sun. This angle, measured 0 to 360 degrees eastward from the
reference vector, changes as a function of the day of the year for a given right
ascension and declination, as the spacecraft orientation must keep the solar
arrays optimally facing the sun.
Angles in the FES field of view are also defined with respect to the
reference vector which points to the sun and is parallel to the pitch
direction. When the spacecraft roll is 0 degrees, the reference vector points
north. Due to the odd number of reflections in the optical path to the FES, the
FES image is displayed in a left-handed coordinate system. All angles in the
FES, therefore, increase in a counterclockwise direction. The reference vector
for FES No.2, the system in current use (see Figure 3.3), is rotated
approximately 28.31 degrees in the FES coordinate system from the negative Y
axis toward the negative X axis.
Let the FES position angle in the plane of the sky be defined such that 0
degrees points north and 90 degrees east. [These definitions are slightly
different than those of Schiffer (1980a).] The position angle is related to the
spacecraft roll by:
position angle = aperture orientation angle - spacecraft roll angle,
where the orientation angles of the apertures are defined with respect
to the reference vector and increase counterclockwise from the reference vector.
The aperture orientation angles are given in Table 3.2.
| Aperture | Orientation Angle | Separation |
| | | |
| LWLA major axis | 73° ± 3° | -- |
| SWLA major axis | 73° ± 2° | -- |
| LWLA to LWSA | 238° | 42.7 arc seconds |
| SWLA to SWSA | 242° | 39.9 |
| LWLA to SWLA | 275° | 66.3 |
| LWSA to SWSA | 279° | 66.2 |
| | |
Table 3.2: IUE Aperture Plate Orientation Angles
The orientation of the apertures relative to the FES coordinate system is given
in Figure 3.3. The orientation of the field as projected on the camera
faceplates may also be determined. The north direction in the FES image is,
therefore, rotated counterclockwise from the reference vector by an amount equal
to the spacecraft roll angle (see Figure 3.3). Figure 3.4 shows the placement
of the large and small apertures with respect to low-dispersion LWP, LWR and SWP
spectra. (The apertures have been enlarged for clarity.) While the orientation
of the FES coordinate system is different for each camera, the dispersion
direction is approximately aligned with the FES Y axis in low dispersion and
with the FES X axis in high dispersion.
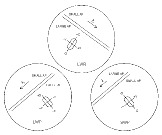 Figure 3.4: Aperture orientation on
the camera faceplates. The locations of low-dispersion spectra for the large
and small apertures are shown for the LWP, LWR, and SWP cameras. The projection
of the large aperture is also indicated for each camera. FES No.2 X and Y
directions are displayed relative to the apertures and spectra. The relative
sizes and angles in this figure are for illustration only.
Figure 3.4: Aperture orientation on
the camera faceplates. The locations of low-dispersion spectra for the large
and small apertures are shown for the LWP, LWR, and SWP cameras. The projection
of the large aperture is also indicated for each camera. FES No.2 X and Y
directions are displayed relative to the apertures and spectra. The relative
sizes and angles in this figure are for illustration only.
The spacecraft roll angle can be obtained from GSFC scripts for images after LWR
6528 and SWP 7549, listed as "S/C ROLL". For those scripts where the roll is
not given, it may be calculated by the method given below. Note: there is
another roll angle ("FSS ROLL") written on the scripts. This is the roll with
respect to the sun and is not equivalent to the spacecraft roll angle.
Calculation of the spacecraft roll and Beta angles for a specific date requires
the target and solar equatorial coordinates (precessed to 1950.0) and are
given by the following equations:
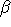 =
cos¯¹(-sin
=
cos¯¹(-sin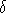 sin
sin![(Dec[Sun])](img23.gif) - cos
- cos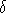 cos
cos![(Dec[Sun])](img23.gif) cos(
cos( -
- ![RA[Sun]](img22.gif) ))
))
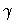 =
cos¯¹(-sin
=
cos¯¹(-sin![(Dec[Sun])](img23.gif) /(sin
/(sin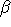 cos
cos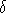 )
- tan
)
- tan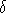 /tan
/tan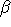 )
)
If sin( -
-
![RA[Sun]](img22.gif) ) < 0,
then S/C roll = 180 +
) < 0,
then S/C roll = 180 + 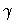
If sin( -
-
![RA[Sun]](img22.gif) ) > 0,
then S/C roll = 180 -
) > 0,
then S/C roll = 180 - 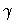
where
![RA[Sun]](img22.gif) = Right
ascension of the sun
= Right
ascension of the sun
![Dec[Sun]](img23.gif) =
Declination of the sun
=
Declination of the sun
 = Right ascension
of the target
= Right ascension
of the target
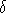 = Declination of
the target.
= Declination of
the target.
An example of this calculation for 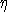 UMa on July 2, 1985 is given below.
UMa on July 2, 1985 is given below.
The sun's position on July 2, 1985 at 00:00 UT was
![RA[Sun]](img22.gif) =
06h41m39.4s,
=
06h41m39.4s,
![Dec[Sun]](img23.gif) =
23°05'37''(1950)
=
23°05'37''(1950)
The target 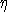 UMa
is at
UMa
is at
 =
13h45m34.3s,
=
13h45m34.3s,
 =
49°33'44''(1950)
=
49°33'44''(1950)
Computed Result: Beta = 97°43'03'', Spacecraft Roll =
63°10'38''.
Thus  UMa was in
a good Beta angle region for battery power.
North is thus 63 degrees counterclockwise from the reference vector. In
this case the large apertures are oriented along a line running from NE
to SW. For a given camera, the small aperture is located south of the
large aperture.
UMa was in
a good Beta angle region for battery power.
North is thus 63 degrees counterclockwise from the reference vector. In
this case the large apertures are oriented along a line running from NE
to SW. For a given camera, the small aperture is located south of the
large aperture.
The major axes of the SWLA and LWLA are oriented 10 degrees East of North.
The LWSA is 175 degrees East of North from the LWLA.
The SWSA is 179 degrees East of North from the SWLA.
The SWLA is 32 degrees West of South from the LWLA.



Next: 3.9 Choice of Long-Wavelength Camera
Up: 3 Pre-Observation Preparations
Previous: 3.7 Moving Targets
Last updated: 15 July 1997
jrc
 Figure 3.3: Aperture plate as
viewed by FES
No. 2. The locations of the apertures and the reference point are shown as
seen by FES No.2. The direction of the reference vector (the direction of
stellar motion for a positive pitch slew) is indicated. The X and Y FES
coordinates are given in FES fine units (1 unit = 0.26 arcseconds). The North
and East directions are shown for a spacecraft roll of 130 degrees.
Figure 3.3: Aperture plate as
viewed by FES
No. 2. The locations of the apertures and the reference point are shown as
seen by FES No.2. The direction of the reference vector (the direction of
stellar motion for a positive pitch slew) is indicated. The X and Y FES
coordinates are given in FES fine units (1 unit = 0.26 arcseconds). The North
and East directions are shown for a spacecraft roll of 130 degrees.